John Fenton's Homepage -
last updated 2 May 2023
Contact
Home address
St-Ulrichs-Platz 2/4
1070 Vienna
Austria
Phones:
Home +43 1 522 7467
Mobile/Handy/Cellulare:
John +43 664 7313 1035
Cristina +43 650 762 2417
Work
Guest Professor
Institute of Hydraulic Engineering and Water Resources
Management
TU Wien / Vienna University of Technology
Karlsplatz 13/222, 1040 Vienna, Austria
E-mail: john.fenton@tuwien.ac.at
Homepage: https://www.kw.tuwien.ac.at/en/team/visiting-professors/profile/fenton/
Family History
John's mother spent many years researching the Family History
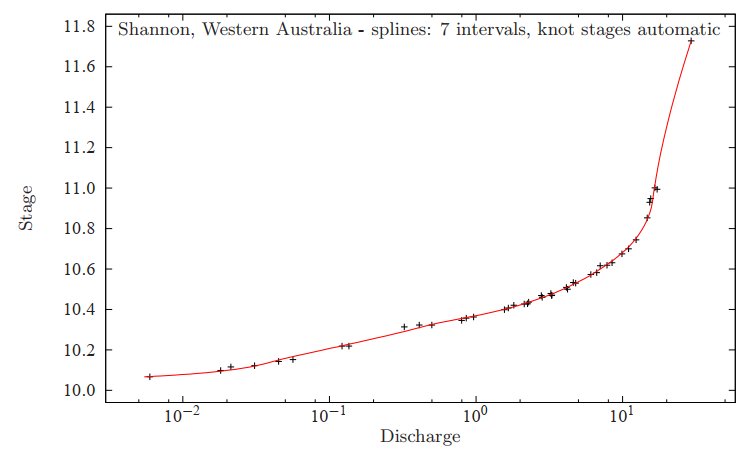
A computer program for calculating rating curves
I have developed a computer program that reads in rating
data for a gauging station or structure and calculates a
rating curve using least squares methods. It has nothing
to do with the old method of trying to fit straight lines
on log-log axes. It seems to work well.
The program and its operation are described here.
All files necessary for the operation of the program are
in http://johndfenton.com/Rating-curves/Program-Files.exe
(it is necessary to copy that link text and paste it into
your internet browser). It is a self-extracting file that,
when downloaded and executed (after your computer maybe
asks you to say that it is acceptable), unpacks the files,
retaining the original file structure, under a directory
of your choice. The program is based on the research
described in two documents:
- A technical report: Fenton, J. D. (2015) Generating
stream rating information from data, Paper 8,
Alternative Hydraulics, and
- A journal paper: Fenton, J. D. (2018) On the generation of stream rating curves, Journal of Hydrology, 564, 748 - 757. https://doi.org/10.1016/j.jhydrol.2018.07.025
In those papers a number of different aspects of the
problem are considered, including calculating Rating
Envelopes for scattered data, and the incorporation
of dates of ratings so that one can calculate a rating
curve for any day in the past.
A method for approximating, smoothing, differentiating, interpolating, or calculating an envelope to data
While working on river rating curves I developed a program using quadratic or cubic splines. Its primary use was to approximate noisy data, but the method seems quite flexible to approximate more-or-less scattered data, or to smooth, differentiate, interpolate, or calculate an envelope to that data. An example is Nikuradse's results for resistance in pipes, which shows variable curvature: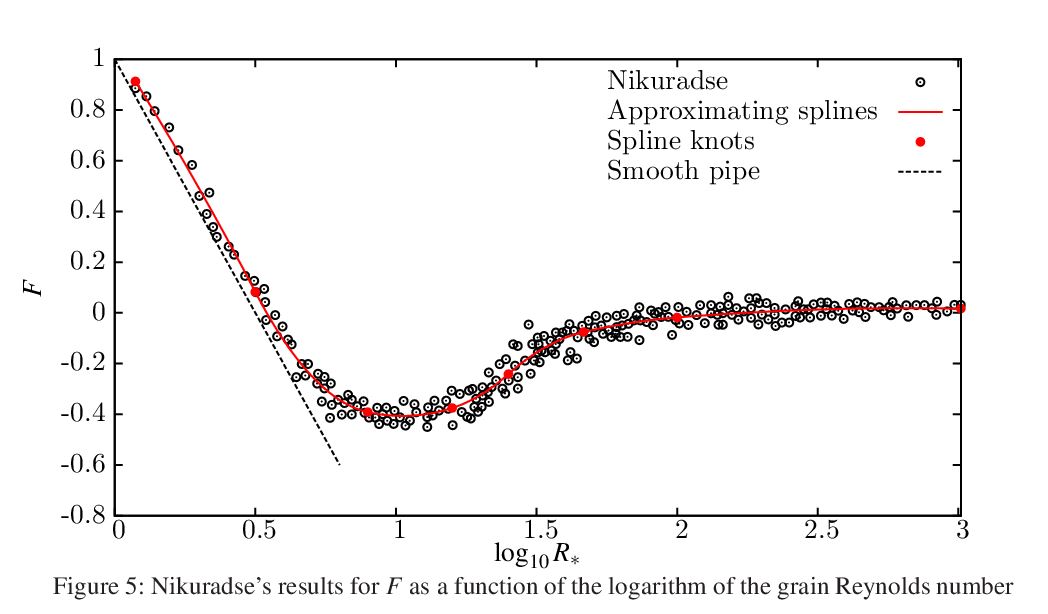
The program and a supporting theoretical document are described here.
Collected papers
Full list of papers
Recent publications
-
Fenton, John D., Huber, Boris, Klasz, Gerhard and Krouzecky, Norbert (2023) Ship waves in rivers: Environmental criteria and analysis methods for measurements,, River Research and Applications 39(4), 629-647.
A literature review shows that the most important physical quantities determining environmental impact of ship waves in a waterway are the fluid velocities, maximum and minimum water levels, and size of drawdown events. Fluid velocity can vary strongly in the vertical so that the usual measurements at a single point are not enough unless made near where the effects are most important, often the bed. Customary use of wave height as a measure of impact has been misleading, because the all-important fluid velocity is of a scale given by wave height divided by wave period. A good and simple estimate of the surface velocity as a disturbance scale is shown to be given by the time derivative of the free surface height. The most important role of linear wave theory is to explain and understand the physics and measurement procedures, such as done here in several places. Its use for obtaining numerical results is criticised. Instead, three integral measures of impact are proposed, all of which use surface elevation measurements and which require no essential mathematical approximations or wave-by-wave analysis. The methods are applied to a study of ship waves on the Danube River. A number of results are presented, including examination of the effects of measurement frequency. After a ship passage, due to repeated shoreline reflections of the wake waves, the river is brought into a long-lasting state of short-crested disturbances, with finite fluid velocities. The environmental consequences of this might be important. After the primary and secondary ship waves it could be called the tertiary wave system.
- Fenton, J. D. (2019) Flood
routing methods, Journal of Hydrology 570,
251-264.
The hierarchy of one-dimensional equations and numerical methods describing the motion of floods and disturbances in streams is studied, critically reviewed, and a number of results obtained. Initially the long wave equations are considered. When presented in terms of discharge and cross-sectional area they enable the development of simple fully-nonlinear advection-diffusion models whose only approximation is that disturbances be very long, easily satisfied in most flood routing problems. Then, making the approximation that changes in surface slope are relatively small such that diffusion terms in the equations are small, various advection-diffusion and Muskingum models are derived. Several well-known Muskingum formulations are tested; one is found to be in error. The three families of the governing equations, the long wave equations, the advection-diffusion and the Muskingum approximations, are linearised and analytical solutions obtained. A dimensionless diffusion-frequency number measures the accuracies of the approximate methods. Criteria for practical use are given, which reveal when they have difficulties for streams of small slope, for fast-rising floods, and/or when shorter period waves are present in an inflow hydrograph. They can probably be used in most flood routing problems with an idealised smooth inflow. However the fact that they cannot be used for all problems requires a general alternative flood routing method, for which it is recommended to use the long wave equations themselves written in terms of discharge and cross-sectional area, when a surprisingly simple physical stream model can be used. An explicit finite difference numerical method is presented that can be used with different inflow specifications and downstream boundary conditions, and is recommended for general use. - Fenton, J. D. (2018) On
the generation of stream rating curves, Journal
of Hydrology 564, 748 - 757.
Traditional methods for the calculation of rating curves from measurements of water level and discharge are criticised as being limited and complicated to implement, such that manual methods are still often used. Two methods for automatic computation are developed using least-squares approximation, one based on polynomials and the other on piecewise-continuous splines. Computational problems are investigated and procedures recommended to overcome them. Both methods are found to work well and once the parameters for a gauging station have been determined, rating data can be processed automatically. For some streams, ephemeral changes of resistance may be important, evidenced by scattered or loopy data. For such cases, the approximation methods can be used to generate a rating envelope as well, allowing the routine calculation also of maximum and minimum expected flows. Criticism is made of current shift curve practices. Finally, the approximation methods allow the specification of weights for the data points, enabling the filtering of data, especially decreasing the importance of points with age and allowing the computation of a rating curve for any time in the past or present. - Fenton, J. D. (2018) Where "Small is Beautiful'' -
Mathematical Modelling and Free Surface Flows, Chapter 3
in
Free Surface Flows and Transport Processes,
Kalinowska, M. B.; Mrokowska, M. M. & Rowiński, P.
M. (Eds.), Proc. 36th International School of
Hydraulics, Jachranka, Poland, May 2017, Springer.
Mathematical and computational models in river and canal hydraulics often require data that may not be available, or it might be available and accurate while other information is only roughly known. There is considerable room for the development of approximate models requiring fewer details but giving more insight. Techniques are presented, especially linearisation, which is used in several places. A selection of helpful mathematical methods is presented. The approximation of data is discussed and methods presented, showing that a slightly more sophisticated approach is necessary. Several problems in waves and flows in open channels are then examined. Complicated methods have often been used instead of standard simple numerical ones. The one-dimensional long wave equations are discussed and presented. A formulation in terms of cross-sectional area is shown to have a surprising property, that the equations can be solved with little knowledge of the stream bathymetry. Generalised finite difference methods for long wave equations are presented and used. They have long been incorrectly believed to be unstable, which has stunted development in the field. Past presentations of boundary conditions have been unsatisfactory, and a systematic exposition is given using finite differences. The nature of the long wave equations and their solutions is examined. A simplified but accurate equation for flood routing is presented. However, numerical solution of the long wave equations by explicit finite differences is also simple, and more general. A common problem, the numerical solution of steady flows is then discussed. Traditional methods are criticised and simple standard numerical ones are proposed and demonstrated. A linearised model for the surface profile of a stream is obtained, also to give solutions without requiring detailed bathymetric knowledge.
- Darvishi, E., Fenton, J. D. and Kouchakzadeh, S.
(2017) Boussinesq
equations for flows over steep slopes and structures,
Journal of Hydraulic Research 55(3),
324-337.
A finite-slope Boussinesq equation is developed to model curved transcritical flow over spillways and broad-crested weirs, even with large slopes. A number of laboratory experiments were performed with different transcritical flow problems including changes in channel gradients and a trapezoidal weir. The equation and the numerical model were tested using results from those experiments and from those for a steep and sharply-curved weir structure, with good results. They can be used as a computational flume to determine the head-discharge characteristics of proposed structures. A novel feature of the equation and numerical method is that higher derivatives of the bed topography are best ignored, apparently mimicking the effects of flow separation in smoothing it. - Fenton, J. D. (2016) Hydraulics: science, knowledge, and culture, Journal of Hydraulic Research 54(5), 485-501.
- Fenton, J. D. and Darvishi, E. (2016) Discussion of "Minimum specific energy and transcritical flow in unsteady open-channel flow" by Oscar Castro-Orgaz and Hubert Chanson, Journal of Irrigation and Drainage Engineering 142(10), http://ascelibrary.org/doi/10.1061/(ASCE)IR.1943-4774.0001077
- Fenton, J. D. (2015) Basic physical processes in
rivers, Chapter 1 in
Rivers – Physical, Fluvial and Environmental
Processes, (eds) Paweł Rowiński and Artur
Radecki-Pawlik, Springer.
The governing long wave equations for a curved channel can be developed with very few limiting approximations, especially using momentum rather than energy. The curvature is then shown to be rarely important and is subsequently ignored. Wave periods, imposed by boundary conditions, are asserted to be fundamental. Long waves have speeds and propagation properties that depend on period, and there is no such thing as a single long wave speed. Examination of dimensionless equations and solution of linearised equations using wave period shows a novel interpretation of terms in the momentum equation: the ”kinematic” approximation and wave are misnomers: the approximation lies not in the neglect of inertial terms but is actually a very long period one. The outstanding problem of river modelling, however, is that of resistance to the flow. A large data set from stream-gaugings is considered and it is shown that the state of the bed, namely the arrangement of bed grains by previous flows, is more important than actual grain size. A formula for resistance is proposed which contains a parameter representing bed state. As that state is usually changing with flow, one can not be sure what the resistance actually will be. This uncertainty may have important implications for modelling. The momentum principle is then applied also to obstacles such as bridge piers, and a simple approximation gives greater understanding and a practical method for incorporation in river models. Finally, river junctions are considered, and the momentum approach with the very long period approximation shows that they can be modelled simply.
The processes of thinking, research, dissemination, and use of research and knowledge in hydraulics are examined, differentiating it from hydrology, and suggesting greater use of scientific methods and theories. At highly technical levels, that is already done, but it is suggested that there is room for a greater simplicity of approach, based on scientific rigour, recognising that much of what is done in hydraulics is modelling. This would make understanding, access to, and participation in research easier for members of the profession. A number of recommendations and conclusions are made. The article has a critical tone, but its main intention is to be helpful to individual hydraulicians and to the profession at large. Suggestions are made as to how the profession might use the Web to give open access to research findings and to create an open resource for hydraulics knowledge, as connection by colleagues in all countries is now possible and feasible.
We show that there is no justification for the Singular Point Theory of Massé (1938) for calculating transitional flows between sub- and super-critical flows.
This was given the 2018 Best Discussion Award by the Journal of Irrigation and Drainage Engineering.
Lectures in hydraulics, numerics, and maritime
engineering
River Engineering
The most recent set of lecture notes here are those for a
course on River Engineering at the Vienna University of
Technology in 2021-2022: Home Page
Lecture notes as slides in colour
Lecture notes for printing in small format, B\&W
A First Course in Hydraulics
This is an introduction to hydraulics. True energy conservation is presented, and Bernoulli's equation is left to a subsidiary (and occasionally useful) role. It is an integrated momentum equation which is valid along a streamline and whose "constant" varies across streamlines. It is absurd to expect to apply it in fully-turbulent three-dimensional situations - for example the flow from a reservoir to a tap in a house. It is much more intellectually honest simply to use conservation of energy, which is more easily derived and is a more plausible model of most hydraulic problems.
Coastal and Ocean Engineering
A final year elective subject, dealing with elementary oceanography, water wave theory, tsunami, and coastal engineering
Computations and Open Channel Hydraulics
An introduction to numerical methods, presenting theory and applications largely using the optimisation package in Microsoft Excel.
Numerical
Methods
This is a set of notes describing the application of
numerical methods. There are some innovations.
Coastal and Ocean Engineering - Steady water waves
A computer program ("Fourier") that solves the problem of
steadily-progressing waves over a flat bottom is described
and made available here: Fourier
Programs that implement Stokes and cnoidal theories are also available. The instructions file for all is Instructions.pdf., which is also included in Fourier.zip. The latest changes are shown as highlighted comments.
The three wave programs are
- A Fourier approximation method whose only approximation lies in truncating the number of terms in the approximating series: Fourier.zip - current version, 23 July 2015.
- An implementation of cnoidal theory, which is based on series expansions in shallowness, requiring that the waves be long relative to the water depth : Cnoidal.zip - current version, 20 March 2015 (to be unpacked in a sub-directory of the Fourier one). This is an approximation, and not as applicable to higher waves as the Fourier method. It can be used as a check on that method - for long waves that are not high, both should give the same results.
- An implementation of Stokes theory, requiring that the waves be not too long relative to the water depth: Stokes.zip - current version 20 March 2015 (to be unpacked in a sub-directory of the Fourier one). This is also an approximation, and not as applicable to higher waves as the Fourier method. It can be used as a check on that method - for waves that are not high or long, both should give the same results.
Maintained and authorised by John Fenton;
Last modified: 2 May 2023